Summer 2013
MATH 225: Multivariable Calculus and Geometry. II
Branko Ćurgus

Wednesday, August 14, 2013

---

• The assigned problems for Section 20.3 are 3, 7, 11, 12, 13, 14, 19, 21, 26, 27, 28, 29, 31, 36.

---

Thursday, August 8, 2013

---

• The assigned problems for Section 20.1 are 1, 2, 5, 7, 9, 12, 17, 19, 20, 21, 23, 29.
• The assigned problems for Section 20.2 are 1, 3, 5, 7, 11, 17, 20, 21, 23, 27, 28, 29, 31, 33, 37, 43.
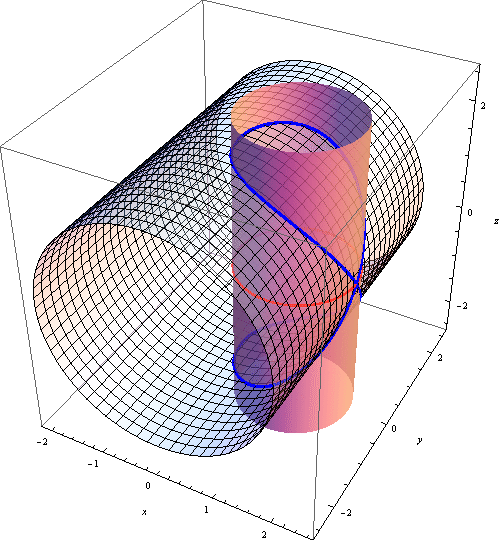
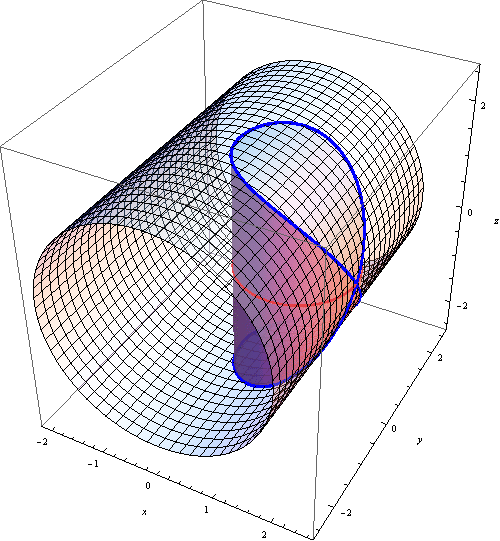
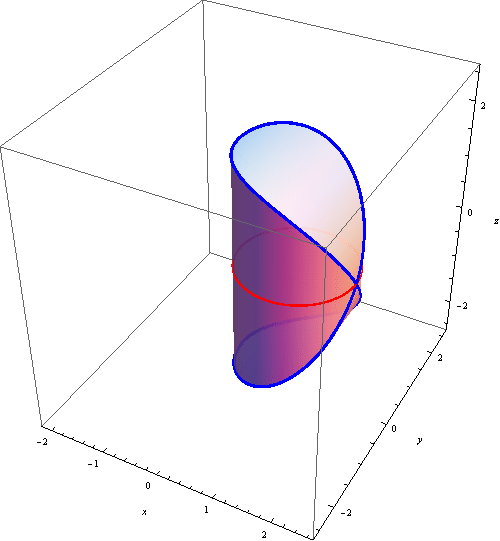
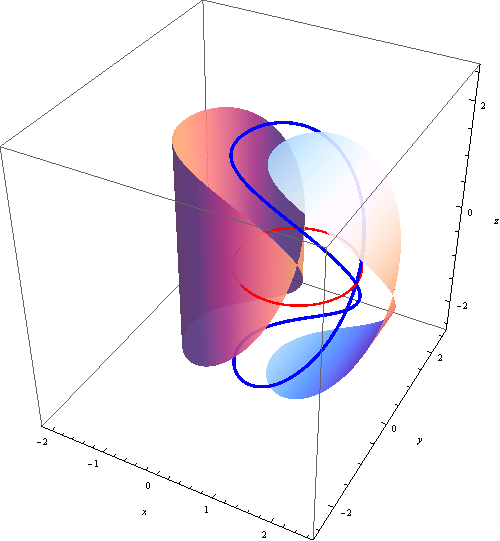
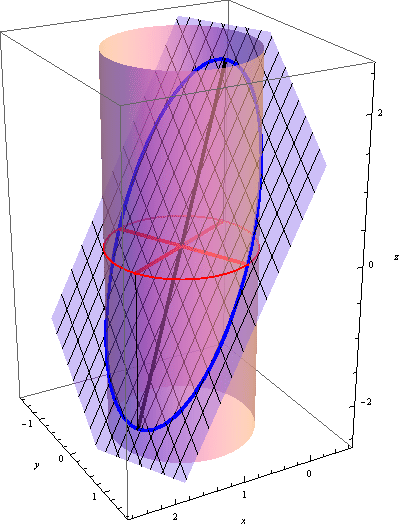
• Yesterday I posted pictures related to an intersection of a cylinder and a plane. That problem reminded me of a problem that I still remember from the time when I was an undergraduate student at the University of Sarajevo.
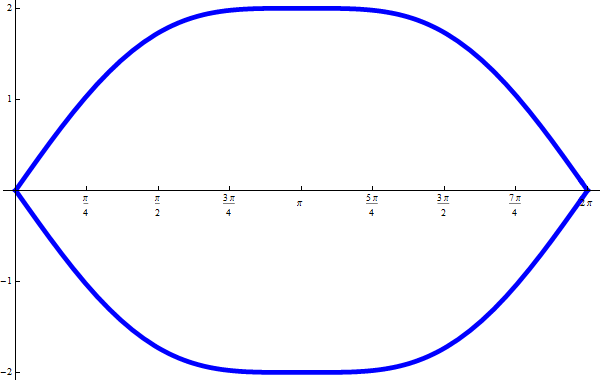
  • The problem related to the intersection of two cylinders. The smaller cylinder is vertical with the equation: $x^2 + y^2 = 2x$ and $z$ is arbitrary. The larger cylinder is horizontal with the equation: $x^2 + z^2 = 4$ and $y$ is arbitrary. I do not recall exactly what I was asked to do with these two cylinders. However, I remember well spending a lot of time trying to visualize the intersection. Below I give four figures that help visualize the intersection.
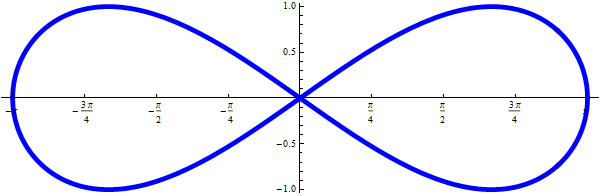
  • The last picture above suggests that two surfaces that enclose the intersections can be unwrapped. Below they are shown unwrapped. The equations of the curves given in the top graph are $y = \sqrt{4- \bigl(1+\cos t \bigr)^2}$ and $y = - \sqrt{4- \bigl(1+\cos t \bigr)^2}$.
    The equations of the curves given in the bottom graph are $y = 2 \sqrt{\bigl(1-\cos(t/2)\bigr)\cos(t/2)}$ and $y = - 2 \sqrt{\bigl(1-\cos(t/2)\bigr)\cos(t/2)}$.
  • None of the calculations related to this intersection are simple enough to be assigned on an exam or even homework. However, Mathematica gives exact results for most calculations.
  • The volume of the intersection of two cylinders is given by the integral \[ 2 \int_0^2 \int_{-\sqrt{2x-x^2}}^{\sqrt{2x-x^2}} \sqrt{4-x^2} \, dy \, dx = 4 \int_0^2 \sqrt{4-x^2} \sqrt{2x-x^2} \, dx = \frac{44}{3}\, \sqrt{2} - 12 \log (1+\sqrt{2}) \approx 10.1653. \]
  • The surface area of the smaller piece can be calculated in two different ways. One way is using the surface integral. \[ 2 \int_0^2 \int_{-\sqrt{2x-x^2}}^{\sqrt{2x-x^2}} \frac{2}{\sqrt{4-x^2}} \, dy \, dx = 8 \int_0^2 \sqrt{\frac{x}{2+x}} \, dx = 16\, \sqrt{2} - 16 \log (1+\sqrt{2}) \approx 8.52544. \] The other way of calculating this area is just integrating the unwrapped function that we pictured above: \[ 4 \int_0^{\pi} 2 \sqrt{\bigl(1-\cos(t/2)\bigr)\cos(t/2)}\, dt = 16\, \sqrt{2} - 16 \log (1+\sqrt{2}) \approx 8.52544. \]
  • The surface area of the lager piece can be calculated by calculating the area of its unwrapped counterpart: \[ 2 \int_0^{2\pi} \sqrt{4- \bigl(1+\cos t \bigr)^2} \, dt = 8\, \sqrt{2} + 4 \log (3+2\sqrt{2}) \approx 18.3647. \]
  • The length of the blue line in all pictures can be calculated in three different ways. First, finding the length of the graph of $y=\sqrt{4- \bigl(1+\cos t \bigr)^2}$: \[ 2 \int_0^{2\pi} \sqrt{1+ \Bigl(\frac{d}{dt} \sqrt{4- \bigl(1+\cos t \bigr)^2} \Bigr)^2} \, dt = 2 \int_0^{2\pi} \sqrt{1+\frac{8 \bigl(\cos(t/2)\bigr)^2}{3+\cos t}}\, dt \approx 15.8856. \] Second, finding the length of the graph of $y = 2 \sqrt{\bigl(1-\cos(t/2)\bigr)\cos(t/2)}$: \[ 4 \int_0^{\pi} \sqrt{1+ \Bigl(\frac{d}{dt} 2 \sqrt{\bigl(1-\cos(t/2)\bigr)\cos(t/2)} \Bigr)^2} \, dt = 2 \int_0^{\pi} \sqrt{3 + 2 \cos t + \frac{1}{\cos(t/2)}} \, dt \approx 15.8856. \] Finally, we can find the length of the blue curve in the first four figures using the parametrization \[ \vec{r}(t) = \Bigl(2 (\cos t )^2, 2(\cos t )(\sin t ), 2\sqrt{1-(\cos t)^4} \Bigr), \qquad -\frac{\pi}{2} \leq t \leq \frac{\pi}{2}. \] Then \[ 2 \int_{-\pi/2}^{\pi/2}\|\vec{r}(t)\|\, dt = 2 \sqrt{2} \int_{-\pi/2}^{\pi/2} \sqrt{ 9 + \cos(4t) - \frac{16}{3+ \cos(2t)} }\,dt \approx 15.8856. \] These three calculations strongly indicate that our calculations for the unwrapped surfaces are correct.

---

Wednesday, August 7, 2013

---

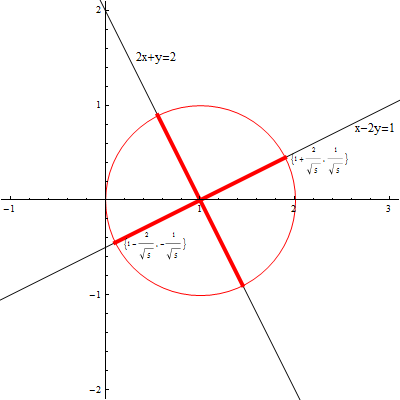
• I want to point out that the area enclosed by the ellipse in Problem 12, Section 19.3 can be evaluated using the formula for the area enclosed by an ellipse that we derived when we studied polar coordinates. This area is $ab\pi$, where $a$ and $b$ are one-half of the ellipse's major and minor axes respectively. To calculate $a$ and $b$ for the ellipse in Problem 12 we proceed as follows.
• The minor axis of the ellipse is the diameter of the circle $x^2+y^2 = 2x$ which is on the line $2x+y=2$ (this line is the intersection of the plane $2x+y+z = 2$ and the $xy$-plane). Thus the minor axis has length $2$.
• The major axis is orthogonal to the minor axes and it is in the plane $2x+y+z = 2$.
• Next, we consider geometric objects in $xy$-plane, see the right figure below. The projection of the major axis onto $xy$-plane is on the line which is orthogonal to the line $2x+y=2$ and goes through the point $(1,0)$. That line is $x-2y=1$. The intersections of this line and the circle $x^2+y^2 = 2x$ are the points $\Bigl(1-\frac{2}{\sqrt{5}},-\frac{1}{\sqrt{5}} \Bigr)$ and $\Bigl(1+\frac{2}{\sqrt{5}},\frac{1}{\sqrt{5}} \Bigr)$. These points are the projections of the endpoints of the major axis onto $xy$-plane.
• Since the end-points of the major axis are in the plane $2x+y+z = 2$, we calculate that the endpoints to be \[ \biggl(1-\frac{2}{\sqrt{5}},-\frac{1}{\sqrt{5}}, \sqrt{5} \biggr), \qquad \biggl(1+\frac{2}{\sqrt{5}},\frac{1}{\sqrt{5}}, -\sqrt{5} \biggr). \] The distance between these two points is $2\sqrt{6}$. Thus the major axis has length $2\sqrt{6}$.
• Thus the area enclosed by the ellipse in Problem 12 is $1\cdot \sqrt{6}\cdot \pi = \pi\sqrt{6}$.

 

---

Tuesday, July 30, 2013

---

• The assigned problems for Section 19.1 are 2, 3, 5, 7, 9, 11, 15, 21, 23, 25, 29, 34, 39, 45.
• The assigned problems for Section 19.2 are 1, 3, 5, 9, 13, 15, 18, 19, 23, 25, 27.
• The assigned problems for Section 19.3 are 1, 3, 5, 7, 8, 9, 10, 11, 12, 15.

---

Monday, July 29, 2013

---

• Exercise 12 in Section 18.4 was not assigned. It is good that we did it in class today. Here are the details. The exercise asks whether the vector field \[ \vec{F}(x,y,z) = 2 x \, \cos(x^2+z^2) \, \vec{i} + \sin(x^2+z^2) \, \vec{j} + 2 z \, \cos(x^2+z^2) \, \vec{k} \] is a gradient field or not. We easily calculated the curl of this filed \[ -2 z \cos(x^2+z^2)\, \vec{i} + 0\, \vec{j} + 2 x \cos(x^2+z^2) \, \vec{k}. \] Thus the curl is not zero. The Curl Test for Vector Fields in 3-space, as stated in our textbook, cannot be used to conclude that $\vec{F}$ is not a gradient filed. However, it should not be too difficult to find a closed curve along which the circulation of $\vec{F}$ is not $0$. Here is one such curve. It consists of four line segments: $C_1$, $C_2$, $C_3$, $C_4$: \begin{alignat*}{3} C_1 \, & : \quad x=t, \quad & & y = 0, \quad & & z=0, \qquad 0\leq t \leq 1; \\ C_2 \, & : \quad x=1, \qquad & & y = t, \quad & & z=0, \qquad 0\leq t \leq 1; \\ C_3 \, & : \quad x=1-t, \quad & & y = 1, \quad & & z=0, \qquad 0\leq t \leq 1; \\ C_4 \, & : \quad x=0, \quad & & y = 1-t, \quad & & z=0, \qquad 0\leq t \leq 1. \\ \end{alignat*} Denote by $C$ the closed curve which is the union of these four line segments. The orientation is implied by the increasing parameter $t$ in the definition. Now we calculate the circulation: \begin{align*} \oint_C \vec{F} \cdot d\vec{r} & = \int_0^1 2t\,\cos(t^2)dt + \int_0^1 \sin(1)dt - \int_0^1 2(1-t)\,\cos((1-t)^2)dt + \int_0^1 0 dt \\ & = \int_0^1 \cos(u)du + \sin(1) + \int_1^0 \cos(u)du \\ & = \sin(1). \end{align*} Since this circulation of $\vec{F}$ is not $0$, the vector field $\vec{F}$ is not a gradient field.
• The assigned problems for Review of Chapter 18 are 14, 15, 17-23, 30, 31, 34, 38, 42, 45, 50.

---

Wednesday, July 24, 2013

---

• The assigned problems for Section 18.4 are 1, 3, 5, 9, 13, 15, 17, 21, 25, 26, 27, 28, 30, 33, 35, 36, 37.

---

Friday, July 19, 2013

---

• Yesterday we started Section 18.3. The assigned problems for 18.3 are 1, 3, 4, 5, 6, 7, 8, 9, 11, 12, 13, 17, 19, 21, 22, 25, 33, 34, 35, 36, 38, 39, 40, 43, 45.
• We still need to do few problems from Section 18.2.

---

Tuesday, July 16, 2013

---

• The assigned problems for 18.1 are 1, 3, 5, 7, 9, 15, 17, 19, 21, 32, 33, 34, 35, 39, 40, 41.
• The assigned problems for 18.2 are 3, 7, 9, 13, 17, 23, 25, 29, 31, 33, 35.

---

Friday, July 12, 2013

---

• I apologize that I did not post any problems from Section 16.7. We did several problems in class and this section will definitely appear on the exam. The assigned problems for 16.7 are 2, 3, 5, 9, 11, 12, 15, 16, 21, 22, 23, 24, 25, 26.

---

Tuesday, July 9, 2013

---

• We started Section 17.5 today. The assigned problems for 17.5 are 1, 3, 5, 15, 17, 19, 25, 28, 29, 30, 34, 37, 39.
• In class I demonstrated plotting of vector fields using the file posted yesterday. I added the part with the numerical computation of a flow line. I also demonstrated relation between parameterized curves and parameterized surfaces. Here is the Mathematica file that I created. It is called Surfaces_2013.nb. As before, right-click on the underlined word "Here"; in the pop-up menu that appears, your browser will offer you to save the file in your directory. Make sure that you save it with the exactly same name. After saving the file you can open it with Mathematica 5.2.
• Here is a Mathematica file with surfaces that I created last year. It has a detailed explanation of the equation of a torus.
• The assigned problems for Review of Chapter 17 are 7, 9, 11, 12, 25, 26, 27-29, 30, 31, 32, 34, 35, 37, 38, 42, 48, 50, 55, 57, 58.

---

Monday, July 8, 2013

---

• Today we did Section 17.3. The assigned problems for 17.3 are 1-10, 11, 12, 13, 15, 16, 17, 20, 22, 23, 25, 26, 27, 28, 31.
• Today we started 17.4. The assigned problems for 17.4 are 4, 5, 7, 8, 9, 15, 16, 17, 18, 20, 21, 22, 23.
• Here is a Mathematica file which illustrates how to plot vector fields in Mathematica. It is called VectorFields.nb. Right-click on the underlined word "Here"; in the pop-up menu that appears, your browser will offer you to save the file in your directory. Make sure that you save it with the exactly same name. After saving the file you can open it with Mathematica 5.2. You will find Mathematica in Start -> All Programs -> Math Applications -> Mathematica. Open Mathematica first, then open VectorFields.nb from Mathematica. You can execute the entire file by the following menu sequence (in Mathematica): Kernel -> Evaluation -> Evaluate Notebook.
• Mathematica 5.2. is an old version. We also have Mathematica 8. These two versions are not compatible. Here is a version 8 file which illustrates how to plot vector fields in Mathematica. The current version is 9. I do not have access to v9, but it seems to me that 8 and 9 are compatible.

---

Tuesday, July 2, 2013

---

• The assigned problems for 17.1 are 1, 2, 4, 6, 9, 15, 16, 23, 26, 29, 32, 36, 40, 44, 45, 46, 48, 49, 50, 51, 56, 57, 58, 59, 62, 63.
• The assigned problems for 17.2 are 2, 3, 6, 8, 10, 12, 13, 14, 17, 19, 23, 25, 26, 31, 33, 34, 36, 37, 38, 45.

---

Wednesday, June 26, 2013

---

• We reviewed in class the area element in polar coordinates: $dA = r \, d\theta \, d r$.
• We reviewed in class the volume element in cylindrical coordinates: $dV = r \, d\theta \, d r \, dz$.
• The volume element in spherical coordinates is: $dV = \rho^2 (\sin \phi) \, d\theta\, d\phi \, d\rho$. I created the image below to help you understand this formula.
  
  
  
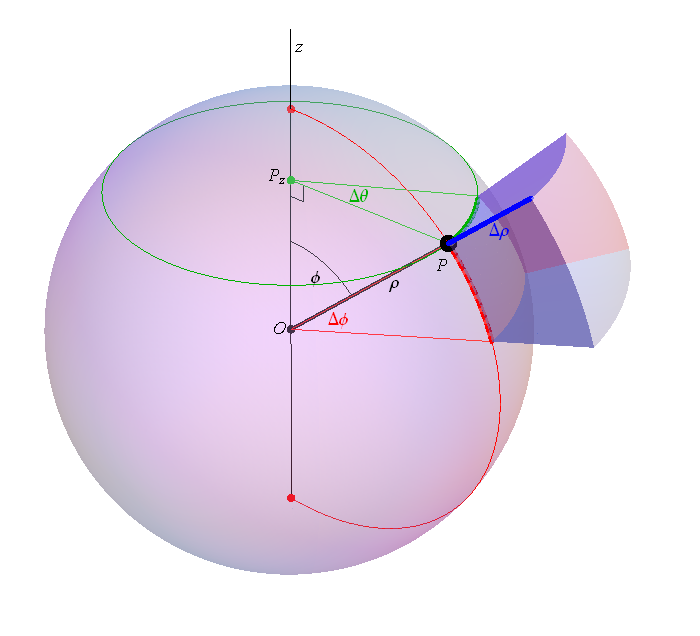
• For the animation of the unit vectors in 3 dimension which can help you understand the spherical coordinates better click here .

---

Tuesday, June 25, 2013

---

• The information sheet
• We will start by reviewing Chapter 16. Here are the problems that I assigned when I taught 224 last time (see Math 224 Winter 2012)
  • The assigned problems for 16.1 are 1, 3, 5, 7, 9-24 (do most), 26, 30, 31.
  • The assigned problems for 16.2 are 1-28 (do most), 31-36, 38, 40, 41, 50-54.
  • The assigned problems for 16.3 are 1-4, 5, 7, 8, 11, 12, 16, 18, 20-23, 25, 27, 32, 35, 36, 37, 38-55, 57, 58.
  • The assigned problems for 16.4 are 1-7, 9, 12-15, 16-19, 20, 22, 24, 25, 27, 28, 29, 31, 32, 33, 35.
  • The assigned problems for 16.5 are 1-20 (all, but in particular 9, 10, 11, 19, 20), 21-35, 36, 37, 38, 40, 42-45, 47, 49-52, 55-58.
  • Some relevant Chapter 16 Review exercises and problems are 14, 19, 20, 48, 49.

---