- I updated a summary of the topics that we covered in this class.
- The assigned problems for Section 20.3 are 3, 7, 11, 12, 13, 14, 19, 21, 26, 27, 28, 29, 31, 36.
- The assigned problems for Section 20.4 are 1, 3, 5, 7, 13, 15, 17, 23, 25, 27, 28, 31.
- The assigned problems for Section 20.1 are 1, 2, 5, 7, 9, 12, 17, 19, 20, 21, 23, 29.
- The assigned problems for Section 20.2 are 1, 3, 5, 7, 11, 17, 20, 21, 23, 27, 28, 29, 31, 33, 37, 43.
- I wrote a summary of the topics that we covered so far.
- Today I did both Section 19.2 and Section 19.3. The principle is the same in both sections. Based on the formula for the surface $S$ we need to calculate the differential area vector $d\vec{A}$. For the surface given as a graph $z = f(x,y)$ the differential vector is \[ d\vec{A} = -f_x \, \vec{\imath} -f_y \, \vec{\jmath} + \vec{k}. \] If the surface $S$ is given by the parametric equations \[ \vec{r}(s,t) = x(s,t) \, \vec{\imath} + y(s,t) \, \vec{\jmath} + z(s,t)\, \vec{k}, \] then \[ d\vec{A} = \vec{r}_s \times \vec{r}_t. \] One has to be careful with this formula and adjust it to match the orientation given in a specific problem.
- The assigned problems for Section 19.2 are 1, 3, 5, 9, 13, 15, 18, 19, 23, 25, 27.
- The assigned problems for Section 19.3 are 1, 3, 5, 7, 8, 9, 10, 11, 12, 15.
-
Here are some problems that you can consider:
- Problem 1. Let \[ \vec{F}(x,y,z) = x \, \vec{\imath} + y\, \vec{\jmath} + z \, \vec{k}. \] Let $S$ be the graph of the function $f(x,y) = 1-x^2-y^2$ defined on the unit circle in the $xy$-plane. Calculate the flux integral of $\vec{F}$ over $S$; that is $\iint_S \vec{F} \cdot d\vec{A}$.
- Problem 2. Let \[ \vec{F}(x,y,z) = x \, \vec{\imath} + y\, \vec{\jmath} + z \, \vec{k}. \] Let $S$ be the closed surface obtained by rotating the graph of the function $y = 1-x^2$ around the $x$-axis. Calculate the flux integral of $\vec{F}$ over $S$; that is $\iint_S \vec{F} \cdot d\vec{A}$.
- Problem 3. Let $S$ be the graph of the function $f(x,y) = 1-x^2-y^2$ defined on the unit circle in the $xy$-plane. Calculate the surface area of $S$.
- The assigned problems for Section 19.1 are 2, 3, 5, 7, 9, 11, 15, 21, 23, 25, 28, 29, 32, 34, 38, 39, 41, 44, 45, 48.
- We finished Section 18.4 today. It is a very interesting section. I tried to clarify the dependence of the Curl Tests for vector fields on the domain. For example, the vector field \[ \vec{F} = -\frac{y}{x^2+y^2} \vec{\imath} + \frac{x}{x^2+y^2} \vec{\jmath} \] is not a gradient field over its domain \[ D = \bigl\{(x,y) \in {\mathbb R}^2 : x^2 + y^2 \neq 0 \bigr\}. \] However, if we restrict the domain to \[ D^\prime = \bigl\{(x,y) \in {\mathbb R}^2 : y \neq 0 \ \text{or} \ x \gt 0 \bigr\}, \] then the vector field $\vec{F}$ is a gradient field. In fact, $\vec{F}$ is the gradient of the function \[ f(x,y) = \begin{cases} -\arctan(x/y) + \pi/2 & \quad \text{if} \quad y \gt 0, \\ \phantom{-}0 & \quad \text{if} \quad y = 0 \quad \text{and} \quad x \gt 0 , \\ -\arctan(x/y) - \pi/2 & \quad \text{if} \quad y \lt 0. \end{cases} \] Notice that the domain of the function $f$ is exactly the set $D^\prime$. Also, notice that $D^\prime$ is the plane from which the origin and the negative $x$-half-axis have been removed.
-
The formula for $f(x,y)$ above looks complicated but this is not too complicated function. Here is its plot.
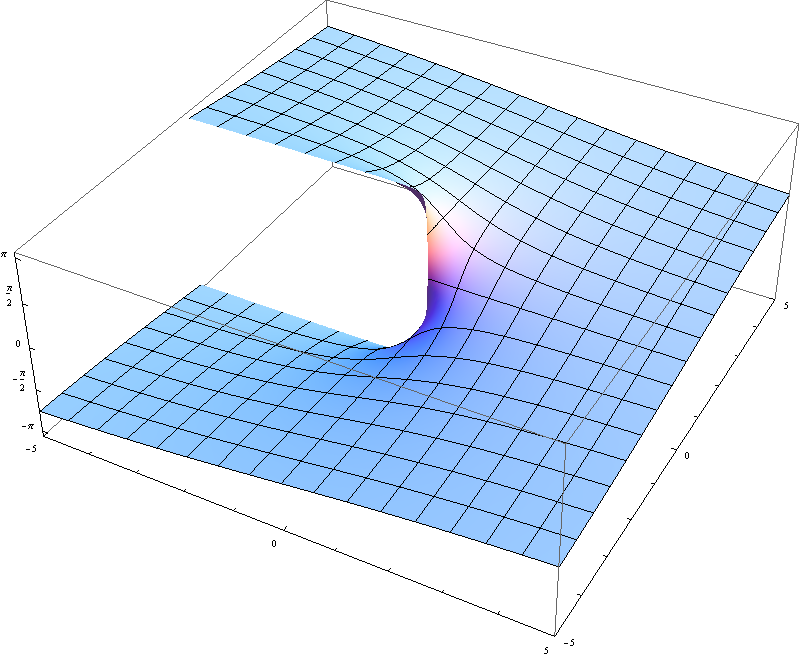
- We did Exercise 12 in Section 18.4. An application of the Curl Test for Vector Fields in 3-space tells us that the vector field in this exercise is not a gradient field. Then I proceeded to find a two curves with the identical endpoints and along which the line integrals differ. I tried two examples and failed to find such curves. Here is one example that you can try: Consider the curve \[ \vec{r}(t) = t \ \vec{\imath} + t^2 \ \vec{\jmath} + 0 \ \vec{k}, \quad 0 \leq t \leq 1, \] from the point $(0,0,0)$ to the point $(1,1,0)$ and the curve \[ \vec{r}(t) = t \ \vec{\imath} + t^4 \ \vec{\jmath} + 0 \ \vec{k}, \quad 0 \leq t \leq 1, \] from the point $(0,0,0)$ to the point $(1,1,0)$. The first line integral evaluates to $1-\cos(1) + \sin(1)$ while the second integral results in $-2\cos(1) + 3 \sin(1)$.
- The assigned problems for Review of Chapter 18 are 14, 15, 17-23, 30, 31, 34, 38, 42, 45, 50.
- Here is the Mathematica file that I created today in class. This file is in version 5.2.
- Today we did Section 18.4. The assigned problems for Section 18.4 are 1, 3, 5, 9, 13, 15, 17, 21, 25, 26, 27, 28, 30, 33, 35, 36, 37.
- Today we did Section 18.3. The assigned problems for 18.3 are 1, 3, 4, 5, 6, 7, 8, 9, 11, 12, 13, 17, 19, 21, 22, 25, 33, 34, 35, 36, 38, 39, 40, 43, 45.
- Today we did Section 18.1. It is important to understand the physical meaning of the line integral as the work done by a force field along a curve. Having this interpretation in mind, one can look at a force field given by its graphical representing and an oriented curve in that field and estimate whether the corresponding work is positive, negative or zero. The assigned problems for 18.1 are 1, 3, 5, 7, 9, 15, 17, 19, 21, 32, 33, 34, 35, 39, 40, 41.
- I did mention how to calculate a simple line integral, so you can try few problems in Section 18.2. The assigned problems for 18.2 are 3, 7, 9, 13, 17, 23, 25, 29, 31, 33, 35.
- I wrote a summary of the topics that we covered so far.
- The first exam is rescheduled for Tuesday, February 3.
-
Thank you for asking Problem 23 from 17.4 today. That is an interesting problem. As stated in the book, the problem is not completely clear. I will restate it to clarify it.
-
Problem 23. Let
\[
\vec{v}(x,y) = (a x - y)\vec{\imath} + (x + a y) \vec{\jmath}
\]
be a vector field and let
\[
\vec{r}(t) = x(t) \vec{\imath} + y(t) \vec{\jmath}
\]
be a flow line of $\vec{v}$.
-
(a) Prove that the function \[ h(t) = e^{-2at}\bigl( \bigl(x(t) \bigr)^2 + \bigl(y(t) \bigr)^2 \bigr) \] is constant. (b) Assume additionally that $\|\vec{r}(0)\| = 1$. Prove that $\|\vec{r}(t)\| = e^{at}$ for all $t$.
-
-
Problem 23. Let
\[
\vec{v}(x,y) = (a x - y)\vec{\imath} + (x + a y) \vec{\jmath}
\]
be a vector field and let
\[
\vec{r}(t) = x(t) \vec{\imath} + y(t) \vec{\jmath}
\]
be a flow line of $\vec{v}$.
- Solution. To prove (a) we differentiate the function $h$. Recall that, since $\vec{r}(t)$ is a flow line of $\vec{v}$ we have \[ x'(t) = a\,x(t) - y(t) \quad \text{and} \quad y'(t) = x(t) + a\, y(t). \] Hence \begin{align*} h'(t) & = -2a \, e^{2at} \bigl( \bigl(x(t) \bigr)^2 + \bigl(y(t) \bigr)^2 \bigr) + e^{2at} \bigl( 2\, x(t) \, x'(t) + 2\, y(t) \, y'(t) \bigr) \\ & = -2a \, e^{2at} \, \bigl( x^2 + y^2 \bigr) + 2\, e^{2at} \, \bigl( x (a\,x - y) + y (x+a\,y) \bigr) \\ & = 0. \end{align*} This proves that $h$ is constant. To prove (b), notice that $\|\vec{r}(0)\| = 1$ means $\bigl(x(0)\bigr)^2 + \bigl(y(0)\bigr)^2 = 1$. Therefore $h(0) = 1$. Since $h$ us constant, we have \[ h(t) = e^{-2at}\bigl( \bigl(x(t) \bigr)^2 + \bigl(y(t) \bigr)^2 \bigr) = 1 \] for all $t$. Hence \[ \bigl(x(t) \bigr)^2 + \bigl(y(t) \bigr)^2 = e^{2at} \] for all $t$. Consequently, \[ \|\vec{r}(t)\| = \sqrt{\bigl(x(t) \bigr)^2 + \bigl(y(t) \bigr)^2} = \sqrt{e^{2at}} = e^{at} \] for all $t$. This proves (b).
- Remark. In Math 331 you learn how to solve \[ x'(t) = a\,x(t) - y(t) \quad \text{and} \quad y'(t) = x(t) + a\, y(t) \] for $x(t)$ and $y(t)$. So, one can find that the solution which satisfies \[ x(0) = x_0 \quad \text{and}\quad y(0) = y_0 \] is \[ x(t) = e^{a t} \bigl( {x_0} \cos(t) - y_0 \sin(t) \bigr) \qquad y(t) = e^{a t} \bigl({x_0} \sin(t) + y_0 \cos(t) \bigr). \] Substituting this solution in the given formula for $h(t)$ and simplifying one can find out that for all $t$ we have \[ h(t) = (x_0)^2 + (y_0)^2. \] This confirms (a).
- Today we did Section 17.4. The assigned problems for 17.4 are 4, 5, 7, 8, 9, 15, 16, 17, 18, 20, 21, 22, 23.
- We will do Section 17.5 tomorrow. The assigned problems for 17.5 are 1, 3, 5, 15, 17, 19, 25, 28, 29, 30, 34, 37, 39.
- The first exam is scheduled for Monday, February 2. I am considering moving the exam to Tuesday, February 3. I will make a formal announcement tomorrow.
- Today we started Section 17.3. The assigned problems for 17.3 are 1-10, 11, 12, 13, 15, 16, 17, 20, 22, 23, 25, 26, 27, 28, 31.
- On Thursday I will show
this Mathematica file which illustrates how to plot vector fields in Mathematica. It is called VectorFields.nb. Right-click on the underlined word "Here"; in the pop-up menu that appears, your browser will offer you to save the file in your directory. Make sure that you save it with the exactly same name. After saving the file you can open it with Mathematica 5.2. You will find Mathematica in
Start -> All Programs -> Math Applications -> Mathematica. Open Mathematica first, then open VectorFields.nb from Mathematica. You can execute the entire file by the following menu sequence (in Mathematica):Kernel -> Evaluation -> Evaluate Notebook. - Mathematica 5.2. is an old version. We also have Mathematica 8. These two versions are not compatible. Here is a version 8 file which illustrates how to plot vector fields in Mathematica.
- More information on how to use Mathematica version 5.2 you can find on my Mathematica 5.2 page. More information on how to use Mathematica version 8 you can find on my Mathematica 8 page.
- Here is the Mathematica file that I created today in class while thinking about Problem 2 from yesterday and Exercise 10 from 17.2. This file is in version 5.2.
- I made a pdf printout of the Mathematica file from the previous item so that you can view it without having access to Mathematica.
-
Below I list few problems in which arc length of a curve can be calculated explicitly.
- Problem 1. Calculate the arc length of the graph of the function $y = x^{3/2}$ between the points $(0,0)$ and $(1,1)$.
- Problem 2. Calculate the arc length of the graph of the function $y= (1/4) x^2-(1/2) \ln x$, between the points $(1,1/4)$ and $\bigl(e,(e^2-2)/4\bigr)$.
- Problem 3. Calculate the arc length of the cycloid given by the parametric equations \[ x(t) = t- \sin(t), \quad y(t) = 1-\cos(t) \quad \text{where} \quad 0 \leq t \leq 2 \pi. \]
- Problem 4. Calculate the arc length of the astroid curve given by the parametric equations \[ x(t) = (\cos t)^3, \quad y(t) = (\sin t)^3 \quad \text{where} \quad 0 \leq t \leq 2 \pi. \]
- Problem 5. Calculate the arc length of the spiral given by the parametric equations \[ x(t) = (\exp t)(\cos t), \quad y(t) = (\exp t)(\sin t) \quad \text{where} \quad -\pi \leq t \leq \pi. \]
- The assigned problems for 17.1 are 1, 2, 4, 6, 9, 14, 15, 16, 23, 26, 27, 29, 32, 36, 40, 44, 45, 46, 48, 49, 50, 51, 56, 57, 58, 59, 62, 63, 64, 68.
- The assigned problems for 17.2 are 2, 3, 6, 8, 10, 12, 13, 14, 17, 19, 23, 25, 26, 31, 33, 34, 36, 37, 38, 45.
- We reviewed the area element in polar coordinates: $dA = r \, d\theta \, d r$.
- We reviewed the volume element in cylindrical coordinates: $dV = r \, d\theta \, d r \, dz$.
-
The volume element in spherical coordinates is: $dV = \rho^2 (\sin \phi) \, d\theta\, d\phi \, d\rho$. I created the image below to help you understand this formula.
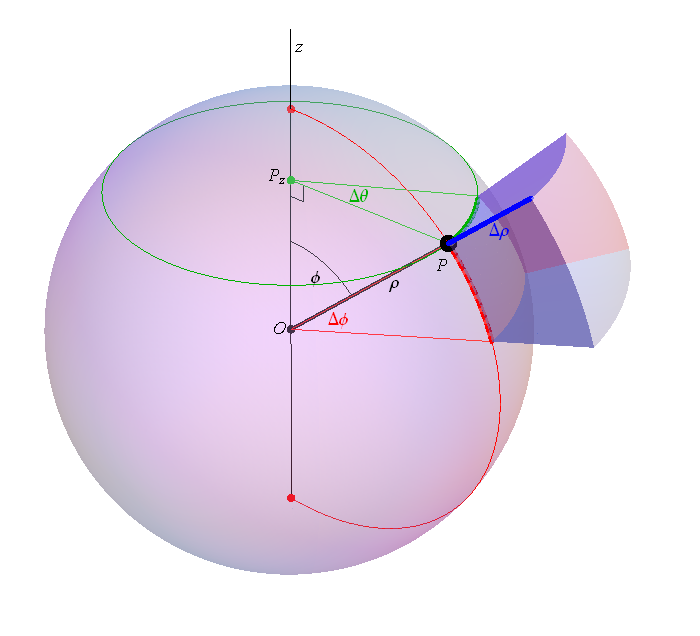
- For the animation of the unit vectors in 3 dimension which can help you understand the spherical coordinates better click here .
- The information sheet
- We will start by reviewing Chapter 16. Here are the problems that I assigned when I taught 224 last time (see Math 224 Winter 2012)
- The assigned problems for 16.1 are 1, 3, 5, 7, 9-24 (do most), 26, 30, 31.
- The assigned problems for 16.2 are 1-28 (do most), 31-36, 38, 40, 41, 50-54.
- The assigned problems for 16.3 are 1-4, 5, 7, 8, 11, 12, 16, 18, 20-23, 25, 27, 32, 35, 36, 37, 38-55, 57, 58.
- The assigned problems for 16.4 are 1-7, 9, 12-15, 16-19, 20, 22, 24, 25, 27, 28, 29, 31, 32, 33, 35.
- The assigned problems for 16.5 are 1-20 (all, but in particular 9, 10, 11, 19, 20), 21-35, 36, 37, 38, 40, 42-45, 47, 49-52, 55-58.
- Some relevant Chapter 16 Review exercises and problems are 14, 19, 20, 48, 49.