- Updated notes on Inner Product Spaces. The second proof of the Cauchy-Bunyakovsky-Schwartz Inequality is now included in the notes.
- I wrote an introduction to Complex Numbers.
-
I hope that the blackboard image from today will help you internalize proof of the Cauchy-Bunyakovsky-Schwartz Inequality based on the Pythagorean Theorem. My impression is that the proof based on the Pythagorean Theorem is easiest to internalize. In the notes I present another proof which I called
"Deus ex machina" proof. My presentation is slightly different from proofs on
Wikipedia . On my webpage Cauchy-Bunyakovsky-Schwarz inequality, I give yet another proof. The proof on this webpage is the only proof that I know which applies to nonnegative "inner products". That is, the proof presented on this websit does not require the definiteness property which is commonly assumed for inner products. On this website, I call such "inner products" nonnegative hermitian sesquilinear forms.
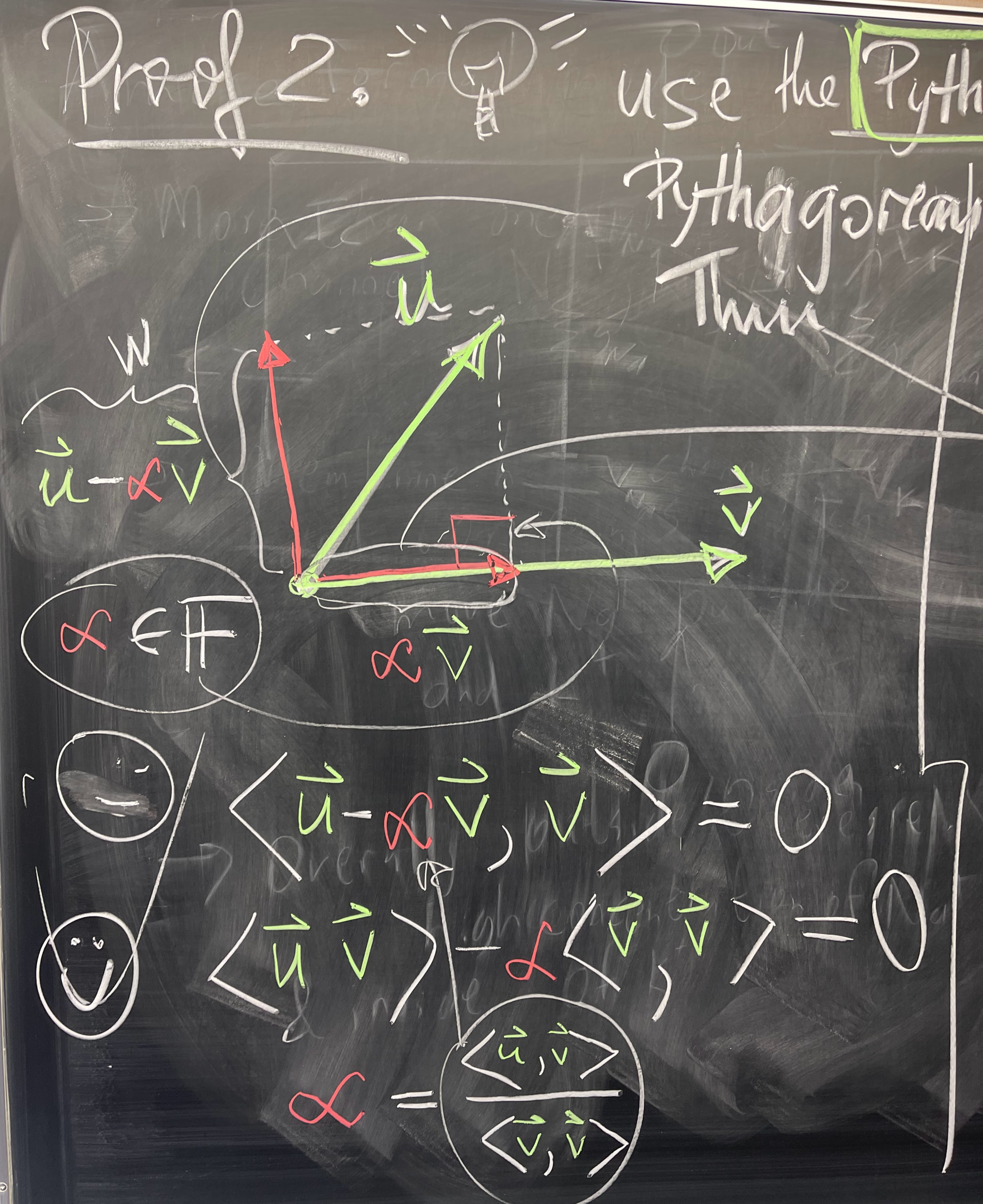
- My notes on Inner Product Spaces. I am revising these notes, so this is work in progress. Up to the Cauchy-Bunyakovsky-Schwartz Inequality the notes follow the presentation in class.
- My notes on Inner Product Spaces. I am revising these notes, so this is work in progress. Up to the Cauchy-Bunyakovsky-Schwartz Inequality the notes follow the presentation in class.
-
In my notes on Eigensystems of Linear Operators, I proved the following two theorems
Theorem. Let $\mathcal{V}$ be a finite-dimensional vector space over a scalar field $\mathbb{F}$ with $\dim\mathcal{V} = n \in \mathbb{N}$. Let $T\in\mathcal{L}(\mathcal{V})$ and assume that there exists a basis $\mathcal{B} = \bigl(v_1,\dots,v_n\bigr)$ of $\mathcal{V}$ for which the matrix $M_{\mathcal{B}}^{\mathcal{B}}(T)$ is upper-triangular with diagonal entries $a_{jj}$ where $j\in \{1,\dots,n\}$. Then $T$ is not injective if and only if there exists $i\in \{1,\dots,n\}$ such that $a_{ii} = 0$.Proof of the first theorem that I gave in class looked simpler than what is in the notes. So, I wrote it down in this file. Proof of the second theorem is the same as in the notes.Theorem. Let $\mathcal{V}$ be a finite-dimensional vector space over a scalar field $\mathbb{F}$ with $\dim\mathcal{V} = n \in \mathbb{N}$. Let $T\in\mathcal{L}(\mathcal{V})$ and assume that there exists a basis $\mathcal{B} = \bigl(v_1,\dots,v_n\bigr)$ of $\mathcal{V}$ for which the matrix $M_{\mathcal{B}}^{\mathcal{B}}(T)$ is upper-triangular with diagonal entries $a_{jj}$ where $j\in \{1,\dots,n\}$. Then \[ \sigma(T) = \bigl\{a_{jj} : j\in \{1,\dots,n\} \bigr\}. \]
-
Now, I am no longer sure why, in an earlier class, I thought to cite
The Road Not Taken by Robert Frost.
The Road Not Taken by Robert Frost
Two roads diverged in a yellow wood, And sorry I could not travel both And be one traveler, long I stood And looked down one as far as I could To where it bent in the undergrowth; Then took the other, as just as fair, And having perhaps the better claim, Because it was grassy and wanted wear; Though as for that the passing there Had worn them really about the same, And both that morning equally lay In leaves no step had trodden black. Oh, I kept the first for another day! Yet knowing how way leads on to way, I doubted if I should ever come back. I shall be telling this with a sigh Somewhere ages and ages hence: Two roads diverged in a wood, and I— I took the one less traveled by, And that has made all the difference.
- Wednesday, February 21, 2024
- In my notes on Eigensystems of Linear Operators, I provided my own proof of the linear independence of eigenvectors corresponding to distinct eigenvalues. Yesterday, in class, I presented a traditional proof using mathematical induction. Here is my write-up of that proof.
- Thursday, February 15, 2024
- My notes on Eigensystems of linear operators.
- Friday, February 9, 2024
- My notes on Linear Operators.
- Tuesday, February 6, 2024 (updated Thursday, February 8, 2024)
-
Yesterday I the coordinate mapping on a finite dimensional vector space by first defining its graph and proving the properties of that graph. It is convenient to introduce the following notational convention. Let $n \in \mathbb{N}$ and let $\mathbb{F}$ be a scalar field. we will use bold case lower case roman letters to denote vectors in $\mathbb{F}^n$ and the components of a named vector in $\mathbb{F}^n$ will be indexed corresponding lower case greek letters. For example for $\mathbf{a} \in \mathbb{F}^n$ the components of $\mathbf{a}$ are $\alpha_1, \ldots, \alpha_n$. That is
\[
\mathbf{a} = \left[\!\begin{array}{c}
\alpha_1 \\ \vdots \\ \alpha_n \end{array}\!\right], \quad
\mathbf{b} = \left[\!\begin{array}{c}
\beta_1 \\ \vdots \\ \beta_n \end{array}\!\right],
\]
and similar. Here is the theorem
Coordination Theorem. Let $n \in \mathbb{N}$ and let $\mathcal{V}$ be a finite dimensional vector space over a scalar field $\mathbb{F}$ with $n = \dim \mathcal{V}$. Let \[ \mathcal{B} = \bigl( b_1, \ldots, b_n \bigr) \in \mathcal{V}^n \] be a basis for $\mathcal{V}$. Prove that the following subset of $\mathcal{V} \times \mathbb{F}^n$ \[ C_{\mathcal{B}} = \left\{ (v, \mathbf{a} ) \in \mathcal{V} \times \mathbb{F}^n \, : \, v = \sum_{k = 1}^{n} \alpha_k b_k \right\} \] is the graph of the bijection $C_{\mathcal{B}} : \mathcal{V} \to \mathbb{F}^n$.
- For the proof we considered the "reversal" of the set $C_{\mathcal{B}}$: \[ \left\{ (\mathbf{a}, v) \in \mathbb{F}^n \times \mathcal{V} \, : \, v = \sum_{k = 1}^{n} \alpha_k b_k \right\} \] and we proved that both, the given set $C_{\mathcal{B}}$ and its reversal are graphs of functions.
- Recall that the columns of the $n\times n$ identity matrix $I_n$ form the standard basis for $\mathbb{F}^n$. This basis I denote by $\mathcal{E}_n$ and its vectors I denote by $\mathbf{e}_1, \ldots, \mathbf{e}_n$: \[ \mathbf{e}_1 = \left[\!\begin{array}{c} 1 \\ 0 \\ \vdots \\ 0 \end{array}\!\right], \quad \mathbf{e}_2 = \left[\!\begin{array}{c} 0 \\ 1 \\ \vdots \\ 0 \end{array}\!\right], \quad \cdots \quad \mathbf{e}_n = \left[\!\begin{array}{c} 0 \\ 0 \\ \vdots \\ 1 \end{array}\!\right]. \] With this notation, our convention for the vectors in $\mathbb{F}$ can be expressed as \[ \mathbf{a} = \sum_{k = 1}^{n} \alpha_k \mathbf{e}_k. \]
-
What I presented in the preceding item, inspired me to give the following definition.
In the definition of $L_{\mathcal{D}}^{\mathcal{C}}$, I use abbreviation s.t. for "such that."Let $\mathcal{V}$ and $\mathcal{W}$ be finite dimensional vector spaces over a scalar field $\mathbb{F}$ and let $m \in \mathbb{N}$. Let \[ \mathcal{C} = \bigl(c_1,\ldots, c_m\bigr) \in \mathcal{V}^m \quad \text{and} \quad \mathcal{D} = \bigl(d_1,\ldots, d_m\bigr) \in \mathcal{W}^m \] be given $m$-tuples. Set \[ L_{\mathcal{D}}^{\mathcal{C}} = \left\{ (v, w ) \in \mathcal{V} \times \mathcal{W} : \exists\mkern 1.5mu \mathbf{a} \in \mathbb{F}^m \ \text{s.t.} \ v = \sum_{k = 1}^{m} \alpha_k c_k \land w = \sum_{k = 1}^{m} \alpha_k d_k \right\}. \]
-
In this item, I list a few problems related to the definition from the previous item. The problems are numbered by upper case roman numerals.
- Problem. Prove that $L_{\mathcal{D}}^{\mathcal{C}}$ is a subspace of $\mathcal{V} \times \mathcal{W}$.
-
Problem. Prove that $L_{\mathcal{D}}^{\mathcal{C}}$ is a graph of a linear operator in $\mathcal{L}(\mathcal{V}, \mathcal{W})$ if and only if $L_{\mathcal{D}}^{\mathcal{C}}$ satisfies two properties of a function
- $\displaystyle \forall\mkern 1.5mu v \in \mathcal{V} \ \ \exists\mkern 1.5mu w \in \mathcal{W}$ such that $(v,w) \in L_{\mathcal{D}}^{\mathcal{C}}$.
- $\displaystyle \forall\mkern 1.5mu (v_1,w_1), (v_2,w_2) \in L_{\mathcal{D}}^{\mathcal{C}}$ we have $v_1 = v_2 \ \ \Rightarrow\ \ w_1 = w_2$.
-
Problem. Find necessary and sufficient conditions on $m\in\mathbb{N}$ and $m$-tuples ${\mathcal{C}}$ and ${\mathcal{D}}$ for $L_{\mathcal{D}}^{\mathcal{C}}$ to be a graph of a linear operator in $\mathcal{L}(\mathcal{V},\mathcal{W})$.
I almost gave up on a necessary and sufficient condition for this problem. But, Ethan suggested that we pursue it, that inspired me to think about this question more, and the below is my contribution.
Solution. To formulate a necessary and sufficient condition, I need to define a new concept. For an $m$-tuple $\mathcal{C} = (c_1,\ldots, c_m) \in \mathcal{V}^m$, define \[ \operatorname{nul}(\mathcal{C}) = \left\{ \mathbf{a} \in \mathbb{F}^m : \sum_{k = 1}^{n} \alpha_k c_k = 0_{\mathcal{V}} \right\}. \] Similarly, for an $m$-tuple $\mathcal{D} = (d_1,\ldots, d_m) \in \mathcal{W}^m$, define \[ \operatorname{nul}(\mathcal{D}) = \left\{ \mathbf{a} \in \mathbb{F}^m : \sum_{k = 1}^{m} \alpha_k d_k = 0_{\mathcal{W}} \right\}. \] With this notation we have: \[ L_{\mathcal{D}}^{\mathcal{C}} \in \mathcal{L}(\mathcal{V}, \mathcal{W}) \quad \Leftrightarrow \quad \mathcal{V} = \operatorname{span}(\mathcal{C}) \ \land \ \operatorname{nul}(\mathcal{C}) \subseteq \operatorname{nul}(\mathcal{D}). \]
In the displayed equivalence we identify an operator with its graph. Therefore, $L_{\mathcal{D}}^{\mathcal{C}} \in \mathcal{L}(\mathcal{V}, \mathcal{W})$ means "$L_{\mathcal{D}}^{\mathcal{C}}$ is a graph of a linear operator."
Comment. Notice that the right-hand side in the displayed equivalence holds when $\mathcal{C}$ is a basis for $\mathcal{V}$ and $\mathcal{D}$ is arbitrary. Also, the right-hand side in the displayed equivalence holds when $\mathcal{V} = \operatorname{span}(\mathcal{C})$ and $\mathcal{D}$ consists of $m$ zeros in $\mathcal{W}$. I noticed both of these facts in class. But I wouldn't have pursued the general case without Ethan's suggestion.
Proof. In Problem II we proved that $L_{\mathcal{D}}^{\mathcal{C}} \in \mathcal{L}(\mathcal{V}, \mathcal{W})$ if and only if the conditions (F1) and (F2) are satisfied. In this proof we will prove that \[ \mathcal{V} = \operatorname{span}(\mathcal{C}) \ \land \ \operatorname{nul}(\mathcal{C}) \subseteq \operatorname{nul}(\mathcal{D}) \quad \Leftrightarrow \quad \text{(F1)} \ \land \ \text{(F2)}. \] In fact, I will prove: \begin{align*} \mathcal{V} = \operatorname{span}(\mathcal{C}) \quad &\Leftrightarrow \quad \text{(F1)}, \\ \operatorname{nul}(\mathcal{C}) \subseteq \operatorname{nul}(\mathcal{D}) \quad &\Leftrightarrow \quad \text{(F2)}. \end{align*} The proof of $\mathcal{V} = \operatorname{span}(\mathcal{C})\ \Leftrightarrow \ \text{(F1)}$ is straightforward.
Next I will prove $\operatorname{nul}(\mathcal{C}) \subseteq \operatorname{nul}(\mathcal{D}) \ \Leftrightarrow \ \text{(F2)}$.
Proof of $\Rightarrow$. Assume $\operatorname{nul}(\mathcal{C}) \subseteq \operatorname{nul}(\mathcal{D})$. I will prove (F2). Let $(v_1,w_1), (v_2,w_2) \in L_{\mathcal{D}}^{\mathcal{C}}$ be arbitrary. By definition of $L_{\mathcal{D}}^{\mathcal{C}}$ this means: \[ \exists\mkern 1.5mu \mathbf{a} \in \mathbb{F}^m \quad \text{s.t.} \quad v_1 = \sum_{k = 1}^{m} \alpha_k c_k \ \land \ w_1 = \sum_{k = 1}^{m} \alpha_k d_k \] and \[ \exists\mkern 1.5mu \mathbf{b} \in \mathbb{F}^m \quad \text{s.t.} \quad v_2 = \sum_{k = 1}^{m} \beta_k c_k \ \land \ w_2 = \sum_{k = 1}^{m} \beta_k d_k. \] To prove $v_1 = v_2 \Rightarrow w_1 = w_2$, assume $v_1 = v_2$. Then \[ v_1 = \sum_{k = 1}^{m} \alpha_k c_k = \sum_{k = 1}^{m} \beta_k c_k = v_2. \] Therefore, \[ \sum_{k = 1}^{m} (\alpha_k - \beta_k) c_k = 0_{\mathcal{V}}, \] that is $\mathbf{a} - \mathbf{b} \in \operatorname{nul}(\mathcal{C})$. Now the assumption $\operatorname{nul}(\mathcal{C}) \subseteq \operatorname{nul}(\mathcal{D})$, yields $\mathbf{a} - \mathbf{b} \in \operatorname{nul}(\mathcal{D})$.By the definition of $\operatorname{nul}(\mathcal{D})$ this means \[ \sum_{k = 1}^{m} (\alpha_k - \beta_k) d_k = 0_{\mathcal{W}}. \] Consequently, \[ w_1 = \sum_{k = 1}^{m} \alpha_k d_k = \sum_{k = 1}^{m} \beta_k d_k = w_2. \] This completes proof of (F2).
Proof of $\Leftarrow$. Assume (F2). I need to prove that $\operatorname{nul}(\mathcal{C}) \subseteq \operatorname{nul}(\mathcal{D})$. Let $\mathbf{a} \in \operatorname{nul}(\mathcal{C})$ be arbitrary. That is assume that \[ \sum_{k = 1}^{m} \alpha_k c_k = 0_{\mathcal{V}}. \] Set \[ w = \sum_{k = 1}^{m} \alpha_k d_k. \] By the definition of $L_{\mathcal{D}}^{\mathcal{C}}$, I deduce that $(0_{\mathcal{V}},w) \in L_{\mathcal{D}}^{\mathcal{C}}$. Clearly $(0_{\mathcal{V}},0_{\mathcal{W}}) \in L_{\mathcal{D}}^{\mathcal{C}}$. Now I apply (F2) with \[ v_1 = 0_{\mathcal{V}}, \quad v_2 = 0_{\mathcal{V}}, \quad w_1 = w, \quad w_2 = 0_{\mathcal{W}}. \] By (F2), I deduce $w = 0_{\mathcal{W}}$. Hence, \[ 0_{\mathcal{W}} = w = \sum_{k = 1}^{m} \alpha_k d_k. \] By the definition of $\operatorname{nul}(\mathcal{D})$, the last equality means $\mathbf{a} \in \operatorname{nul}(\mathcal{D})$. Since $\mathbf{a} \in \operatorname{nul}(\mathcal{C})$ was arbitrary, this proves \[ \operatorname{nul}(\mathcal{C}) \subseteq \operatorname{nul}(\mathcal{D}). \]
Proof is complete. - Problem. Find necessary and sufficient conditions on $m\in\mathbb{N}$ and $m$-tuples ${\mathcal{C}}$ and ${\mathcal{D}}$ for $L_{\mathcal{D}}^{\mathcal{C}}$ to be a graph of an isomorphism in $\mathcal{L}(\mathcal{V},\mathcal{W})$.
- Problem. Let $k \in \mathbb{N}$ and let $\mathcal{A} \in \mathcal{V}^k$ and $\mathcal{B} \in \mathcal{W}^k$. Find necessary and sufficient conditions on $\mathcal{A}$, $\mathcal{B}$, $\mathcal{C}$, and $\mathcal{D}$ so that the sum \[ L_{\mathcal{B}}^{\mathcal{A}} + L_{\mathcal{D}}^{\mathcal{C}} \] is direct.
- Problem. Let $k \in \mathbb{N}$ and let $\mathcal{A} \in \mathcal{V}^k$ and $\mathcal{B} \in \mathcal{W}^k$ be given. Find $l \in\mathbb{N}$ and $\mathcal{X} \in \mathcal{V}^l$ and $\mathcal{Y} \in \mathcal{W}^l$ such that \[ L_{\mathcal{B}}^{\mathcal{A}} + L_{\mathcal{D}}^{\mathcal{C}} = L_{\mathcal{Y}}^{\mathcal{X}}. \] is direct.
- Problem. Find $m \in\mathbb{N}$ and $m$-tuples ${\mathcal{C}}$ and ${\mathcal{D}}$ such that \[ \bigl\{ (0_{\mathcal{V}}, 0_{\mathcal{W}} ) \bigr\} = L_{\mathcal{D}}^{\mathcal{C}}. \]
- Problem. Find $m \in\mathbb{N}$ and $m$-tuples ${\mathcal{C}}$ and ${\mathcal{D}}$ such that \[ \mathcal{V} \times \mathcal{W} = L_{\mathcal{D}}^{\mathcal{C}}. \]
- Problem. Let $\mathcal{F}$ be a subspace of $\mathcal{V}$ and let $\mathcal{G}$ be a subspace of $\mathcal{W}$. Find $m \in\mathbb{N}$ and $m$-tuples $\mathcal{C}$ and ${\mathcal{D}}$ such that \[ \mathcal{F} \times \mathcal{G} = L_{\mathcal{D}}^{\mathcal{C}}. \]
- Problem. Prove that for every subspace $\mathcal{H}$ of $\mathcal{V} \times \mathcal{W}$ there exist $m\in\mathbb{N}$ and $m$-tuples ${\mathcal{C}}$ and ${\mathcal{D}}$ such that $\mathcal{H} = L_{\mathcal{D}}^{\mathcal{C}}$.
- Thursday, February 1, 2024
- Inspired by your questions, I rewrote Problem 7.5 in my notes Vector Spaces. As you have probably noticed, the old version of Problem 7.5 is Problem 3 on Assignment 1. The new version Problem 7.5 will give you some hints towards solving parts (c) and (d) of Problem 3 on Assignment 1.
- Tuesday, January 30, 2024
- Let $\mathbb{F}$ be a scalar field and let $D$ be an infinite subset of $\mathbb{F}$. Today I started proof of the fact that the monomials are linearly independent as functions in the vector space $\mathbb{F}^D$. Since I consider this proof very important, I wrote all the details of the proof in my notes on Bases. This might be the simplest proof of this fact. And my proof contains all the details, does not use any background knowledge except basic algebra.
- Sunday, January 28, 2024 (updated)
-
Here I present remarkable example of a proof using the Principle of Mathematical Induction. This proof is Example 4.5 in the book:
Titu Andreescu, Vlad Crsan Mathematical Induction: A Powerful and Elegant Method of Proof. First recall the exact version of the Principle of Mathematical Induction that we are using.Principle of Mathematical Induction. By $\mathbb{N}$ we denote the set of positive integers. Let $P(n)$ be a propositional function with $n\in \mathbb{N}$. The following implication holds: \[ P(1) \land \Bigl( \forall \mkern 2mu k \in \mathbb{N} \ \ P(k) \Rightarrow P(k+1) \Bigr) \quad \Rightarrow \quad \forall \mkern 2mu n \in \mathbb{N} \ \ \ P(n) \] -
Proof.Problem. By $\mathbb{R}_+$ we denote the set of positive real numbers. For $n \in \mathbb{N}$ by $\mathbb{R}_+^n$ we denote the set of all $n$-tuples of positive real numbers. Prove that the following implication holds for all $n\in\mathbb{N}$: \[ \forall \mkern 2mu (a_1,\ldots,a_n) \in \mathbb{R}_+^n \qquad \prod_{i=1}^n a_i = 1 \quad \Rightarrow \quad n \leq \sum_{i=1}^n a_i . \]
- Let $n\in\mathbb{N}$. Denote the displayed implication in the preceding boxed statement by $P(n)$.
- Base case. The statement $P(1)$ reads: \[ \forall \mkern 1mu a_1 \in \mathbb{R}_+ \qquad a_1 = 1 \quad \Rightarrow \quad a_1 = 1. \] The preceding implication is clearly true.
- Inductive step. Let $k\in\mathbb{N}$ be arbitrary. We need to prove \[ P(k) \quad \Rightarrow \quad P(k+1). \] Assume $P(k)$. That is assume \[ \forall \mkern 2mu (b_1,\ldots,b_k) \in \mathbb{R}_+^k \qquad \prod_{i=1}^k b_i = 1 \quad \Rightarrow \quad k \leq \sum_{i=1}^k b_i. \] The preceding implication is the Inductive hypothesis. We will prove $P(k+1)$. That is we will prove \[ \forall \mkern 2mu (a_1,\ldots,a_{k+1}) \in \mathbb{R}_+^{k+1} \qquad \prod_{i=1}^{k+1} a_i = 1 \quad \Rightarrow \quad k+1 \leq \sum_{i=1}^{k+1} a_i. \] Let $(a_1,\ldots,a_{k+1}) \in \mathbb{R}_+^{k+1}$ be arbitrary and ssume \[ \prod_{i=1}^{k+1} a_i = 1. \] Since neither product, nor sum depend on the order of the $(k+1)$-tuple, we can also assume that \[ \forall \mkern 1mu i \in \{1,\ldots,k+1\} \quad a_1 \leq a_i \leq a_{k+1}. \] With this order we have \[ a_{k+1} \lt 1 \quad \Rightarrow \quad \prod_{i=1}^{k+1} a_i \lt 1. \] Therefore, the contrapositive yields that $a_1\cdots a_{k+1} = 1$ implies $a_{k+1} \geq 1$. Similarly, the assumed order yields \[ a_{1} \gt 1 \quad \Rightarrow \quad \prod_{i=1}^{k+1} a_i \gt 1. \] Therefore, the contrapositive yields that $a_1\cdots a_{k+1} = 1$ implies $a_1 \leq 1$. Notice that \begin{align*} a_1 \leq 1 \ \land \ a_{k+1} \geq 1 &\quad \Rightarrow \quad \bigl(1-a_1\bigr) \bigl(1-a_{k+1}\bigr) \leq 0 \\ &\quad \Rightarrow \quad a_1 a_{k+1} \leq a_1 + a_{k+1} - 1. \end{align*} Now we use the Inductive hypothesis. Let $k$-tuple $(b_1,\ldots,b_k)$ consist of the product $b_1 = a_1a_{k+1}$ and, if $k \gt 1$, $b_i = a_i$ for all $i \in \{2,\ldots,k\}$. Since \[ \prod_{i=1}^k b_i = \prod_{i=1}^{k+1} a_i = 1, \] we can apply the Inductive hypothesis and deduce that \[ k \leq \sum_{i=1}^k b_i. \] Since we proved that \[ b_1 = a_1 a_{k+1} \leq a_1 + a_{k+1} - 1, \] we deduce that \[ k \leq \sum_{i=1}^k b_i = a_1a_{k+1} + \sum_{i=2}^k a_i \leq a_1 + a_{k+1} - 1 + \sum_{i=2}^k a_i. \] Thus, \[ k+1 \leq \sum_{i=1}^{k+1} a_i. \]
-
For the sake of completeness, in the above problem, we should add the condition for the equality to hold in the inequality.
Problem. By $\mathbb{R}_+$ we denote the set of positive real numbers. For $n \in \mathbb{N}$ by $\mathbb{R}_+^n$ we denote the set of all $n$-tuples of positive real numbers. Prove that the following implication holds for all $n\in\mathbb{N}$: \[ \forall \mkern 2mu (a_1,\ldots,a_n) \in \mathbb{R}_+^n \qquad \prod_{i=1}^n a_i = 1 \quad \Rightarrow \quad n \leq \sum_{i=1}^n a_i . \] The equality in the above inequality holds if and only if $(a_1,\ldots,a_n) = (1,\ldots,1)$.
-
An application of the above problem provides one of the simplest proofs of the Inequality of Arithmetic and Geometric Means, see the Wikipedia page AM-GM Inequality
Proof.AM-GM Inequality By $\mathbb{R}_+$ we denote the set of positive real numbers. For $n \in \mathbb{N}$ by $\mathbb{R}_+^n$ we denote the set of all $n$-tuples of positive real numbers. Prove that the following implication holds for all $n\in\mathbb{N}$: \[ \forall\mkern 2mu (x_1,\ldots,x_n) \in \mathbb{R}_+^n \qquad \sqrt[n]{x_1 \cdots x_n} \leq \frac{x_1 + \cdots + x_n}{n}. \] The equality holds if and only if $x_1 = \cdots = x_n$.
- Let $n\in\mathbb{N}$ and $(x_1,\ldots,x_n) \in \mathbb{R}_+^n$ be arbitrary. Set $\alpha = \sqrt[n]{x_1 \cdots x_n}$. Then $\alpha \gt 0$ and $\alpha^n = x_1 \cdots x_n$. Set \[ \forall\mkern 1mu i \in \{1,\ldots,n\} \quad a_i = \frac{x_i}{\alpha}. \] Then, \[ (a_1,\ldots,a_n) \in \mathbb{R}_+^n \ \ \land \ \ \prod_{i=1}^n a_i = \frac{x_1 \cdots x_n}{\alpha^n} = 1. \] By the above Problem we deduce that \[ n \leq \sum_{i=1}^n a_i = \frac{1}{\alpha}\bigl( x_1 + \cdots + x_n \bigr). \] Consequently, since $n \gt 0$ and $\alpha \gt 0$, we have \[ \sqrt[n]{x_1 \cdots x_n} = \alpha \leq \frac{1}{n}\bigl( x_1 + \cdots + x_n \bigr). \] This proves the AM-GM inequality.
-
We started with a problem which is a special case of the AM-GM Inequality, then we used that problem to prove the AM-GM Inequality. We could have chosen a different special case of the AM-GM Inequality and formulated it as a problem. The proof is probably similar.
Problem. By $\mathbb{R}_+$ we denote the set of positive real numbers. For $n \in \mathbb{N}$ by $\mathbb{R}_+^n$ we denote the set of all $n$-tuples of positive real numbers. Prove that the following implication holds for all $n\in\mathbb{N}$: \[ \forall \mkern 2mu (a_1,\ldots,a_n) \in \mathbb{R}_+^n \qquad \sum_{i=1}^n a_i = n \quad \Rightarrow \quad \prod_{i=1}^n a_i \leq 1. \] The equality in the above inequality holds if and only if $(a_1,\ldots,a_n) = (1,\ldots,1)$.
-
Here is a variation on the above problems with an "if and only if" statement:
Problem. By $\mathbb{R}_+$ we denote the set of positive real numbers. For $n \in \mathbb{N}$ by $\mathbb{R}_+^n$ we denote the set of all $n$-tuples of positive real numbers. Prove that the following implication holds for all $n\in\mathbb{N}$: \[ \forall \mkern 2mu (a_1,\ldots,a_n) \in \mathbb{R}_+^n \qquad \prod_{i=1}^n a_i \lt 1 \quad \Leftrightarrow \quad \sum_{i=1}^n a_i \lt n. \]
- Thursday, January 25, 2024
- Today we did the Steinitz Exchange Lemma. The proof in the class notes is by Mathematica Induction. It is quite complicated proof by Mathematical Induction.
-
Below I give a formal statement of the Principle of Mathematical Induction. The Principle of Mathematical Induction is a consequence of the Well Ordering Axiom of Integers.
Principle of Mathematical Induction. Let $\mathbb{N}_0 = \{0\} \cup \mathbb{N}$. Let $P(n)$ be a propositional function with $n\in \mathbb{N}_0$. The following implication holds: \[ P(0) \land \Bigl( \forall \mkern 2mu k \in \mathbb{N}_0 \ \ P(k) \Rightarrow P(k+1) \Bigr) \quad \Rightarrow \quad \forall \mkern 2mu n \in \mathbb{N}_0 \ \ \ P(n) \]
- Friday, January 19, 2024
- We continued discussing Section 2A: Span and Linear Independence in the textbook. See the post on Tuesday.
- In class we briefly discussed the vector space $\mathbb{R}^{\mathbb{N}}$ over the scalar field $\mathbb{R}$. I added Problem 5.1 in my notes Bases about this space. This addition bumped the challenging problem in Bases to Problem 5.3.
- Tuesday, January 16, 2024
- Example 1.44 on page 22 in the textbook is relevant for understanding of direct sums of three subspaces. See also the first paragraph on page 24.
- Exercise 24 on page 24 in the textbook is a relevant exercise about direct sums. Problem 7.5 in my notes Vector Spaces is inspired by Exercise 24.
- Read Section 2A: Span and Linear Independence. Do Exercises 3, 6, 7, 10, 11, 14 in Section 2A. The next section of my notes is entitled Bases. Problem 5.2 in the notes is challenging.
- Friday, January 12, 2024
- I updated my notes on Vector Spaces.
- There are some interesting problems at the end of Vector Spaces.
- Exercise 24 in Section 1C in the textbook is interesting.
- It might be interesting to compare Exercise 8 in Section 1B to Problem 7.10 in my notes Vector Spaces. That is basically the same problem, but written in different style.
- Thursday, January 11, 2024
- I updated my notes on Vector Spaces.
- Rigorous reasoning in Mathematics is based on Mathematical Logic. Therefore it might be a good idea to read my Brief Review of Mathematical Logic. After reading Brief Review of Mathematical Logic you can read my webpage Mathematical Rigor in the Context of Quadratic Functions.
- Tuesday, January 9, 2024 (updated)
-
The reading homework for Thursday:
- Section 1A: Review of complex numbers, the concept of a list (I prefer to call lists tuples), the space $\mathbb{F}^n$ (Notice that in this book the author uses the old-fashioned bold face capitals to denote the scalar fields. I prefer to use, so called blackboldbold font, which seems to be more common nowdays.)
- Section 1B Definition of a vector space and exercises.
- If you find an exercise that you cannot solve please report them in Discussions on Canvas.
- My notes on Vector Spaces.
- My notes on Complex Numbers. There are 11 exercises at the end of my notes. Almost entirely the content of my notes is mentioned in the Common Core State Standards for Mathematics. Some of the exercises are very similar to the exercises mentioned in the Common Core State Standards for Mathematics.
-
I recommend that you look into learning
LaTeX which is a superior typesetting system for writing math papers. Not only that LaTeX is the best way to write mathematical content on computer, but it is the best way to display math on the Internet. All the mathematics that you see on my website is written in LaTeX. All the mathematics that you see on Wikipedia is written in LaTeX. In fact, if you go to a mathematics page, on the line below the title, next to the Read button, you will find the Edit button. Clicking this edit button will take you to the code for that webpage. You will see a lot of LaTeX code there. In fact, if you need exactly that formula, you can copy and paste it in your document.
- I created Getting Started with LaTeX page to help you with this.
- Here is a simple LaTeX sample document in which I prove an interesting inequality.
- If you need help starting with LaTeX, feel free to ask me for help. It is as important as learning one nice piece of mathematics. And I want to help you with that, not only because that is my job, but because I deeply believe that both math - through learning rigorous thinking - and professional writing skills - supported by rigorous thinking - will help you in your life more than anything else.
- I have noticed that many students use the online LaTeX editor Overleaf. I do not have any experience with Overleaf, except that I have seen that many students use it with nice results.
- LaTeX is just like a computer program that compiles into your mathematical document. As you probably heard ChatGPT is exceptionally good at writing code in all kind of different languages. So, it is good at writing LaTeX code. If you are confused on how to do a particular task in LaTeX, you can ask ChatGPT for advise. All advise that I obtained was superb. Not only in LaTeX, but in Wolfram Mathematica as well. Sometimes ChatGPT is sloppy, and makes trivial mistakes, parenthesis not matching and stuff like that. It helps to write very detailed user prompts.
-
You:
Can you please write a complete LaTeX file with instructions on using basic mathematical operations, like fractions, sums, integrals, basic functions, like cosine, sine, and exponential function, and how to structure a document and similar features? Please explain the difference between the inline and displayed mathematical formulas. Please include examples of different ways of formatting displayed mathematical formulas. Please include what you think would be useful to a mathematics student. Also, can you please include your favorite somewhat complicated mathematical formula as an example of the power of LaTeX? I emphasize I want a complete file that I can copy into the LaTeX compiler and compile into a pdf file. Please ensure that your document contains the code for the formulas you are writing, which displays both as code separately from compiled formulas. Also, please double-check that your code compiles correctly. Remember that I am a beginner and cannot fix the errors. Please act as a concerned teacher would do.
This is the LaTeX document that ChatGPT produced base on the above prompt. Here is the compiled PDF document.
You can ask ChatGPT for specific LaTeX advise. To get a good response, think carefully about your prompt. Also, you can offer to ChatGPT a sample of short mathematical writing from the web or a book as a PNG file and it convert that writing to LaTeX. You can even try with neat handwriting. The results will of course depend on the clarity of the file, ChatGPT makes mistakes, but I found it incredibly useful.
- It is tempting to make LaTeX a mandatory tool for creating submissions in this class. However, I do not appreciate having mandates imposed on me; I prefer the freedom to think my own thoughts. An important part of a teacher's role is to encourage students to think their own thoughts, and imposing a mandate could contradict that spirit.
- Friday, December 22, 2023
- Professor Sheldon Axler, the author of Linear Algebra Done Right has made PDF file of the fourth edition of this popular linear algebra book available free of charge. We are taking advantage of this generous gesture and we are using this book this quarter.
- The information sheet (the link will go live before the class starts)
- Some relevant Wikipedia links:
-
Before briefly reflecting on the history of Linear Algebra, I want to celebrate the history of mathematics with one long sentence:
Throughout history, human civilizations continue to develop and share mathematical knowledge; succeeding civilizations recognize and admire the contributions of the preceding ones; prior mathematical creations serve as a foundation and inspiration for further advancements, all in unity, giving mathematics the true spirit of a collective endeavor of the entirety of humanity through history, the present, and the future.
-
What is the oldest linear algebra problem?
-
Clay tablet VAT 8389 from the Old Babylonian period, from 2000 to 1600 BC, contains what is believed to be the earliest word problem that can be interpreted as a system of linear equations:
A translation of this word problem into a system of linear equations is as follows: \begin{alignat*}{4} &x_1 & &\ + &x_2 & = 1800 \\ \tfrac{2}{3} &x_1 & &- \tfrac{1}{2} &x_2 & = \phantom{1}500. \end{alignat*} -
Problem 40 of the Rhind papyrus which is dated to 1550 BC is:
Denote by $x_1$ the smallest number and by $x_2$ the common difference. After simplification the above problem translates into the following system of linear equations: \begin{alignat*}{5} 5 &x_1 & & + 10 &x_2 & = 100 \\ \tfrac{11}{7} &x_1 & & - \phantom{1}\tfrac{2}{7} &x_2 & = \phantom{10}0. \end{alignat*} -
Most importantly for us, the oldest known treatment of systems of linear equations from antiquity which resembles the methods that we will use in this class is in Chapter 8 of the Chinese textbook Nine Chapters of the Mathematical Art which is at least 1800 years old.
-
Clay tablet VAT 8389 from the Old Babylonian period, from 2000 to 1600 BC, contains what is believed to be the earliest word problem that can be interpreted as a system of linear equations:
- If the history of mathematics might inspire you to study mathematics with more enthusiasm, below I link to some websites with more about the history of Linear Algebra.